Questions: Solve the radical equation. Check all proposed solutions. x - sqrt(4x - 19) = 6 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. The solution set is . (Use a comma to separate answers as needed. Simplify your answer.) B. The solution set is ∅.
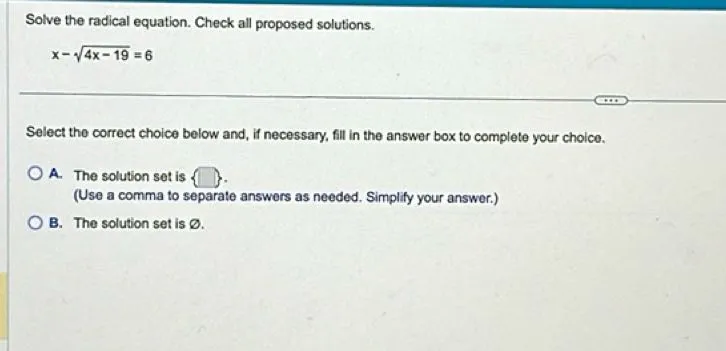
Transcript text: Solve the radical equation. Check all proposed solutions.
\[
x-\sqrt{4 x-19}=6
\]
Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
A. The solution set is $\square$ $\}$.
(Use a comma to separate answers as needed. Simplify your answer.)
B. The solution set is $\varnothing$.





