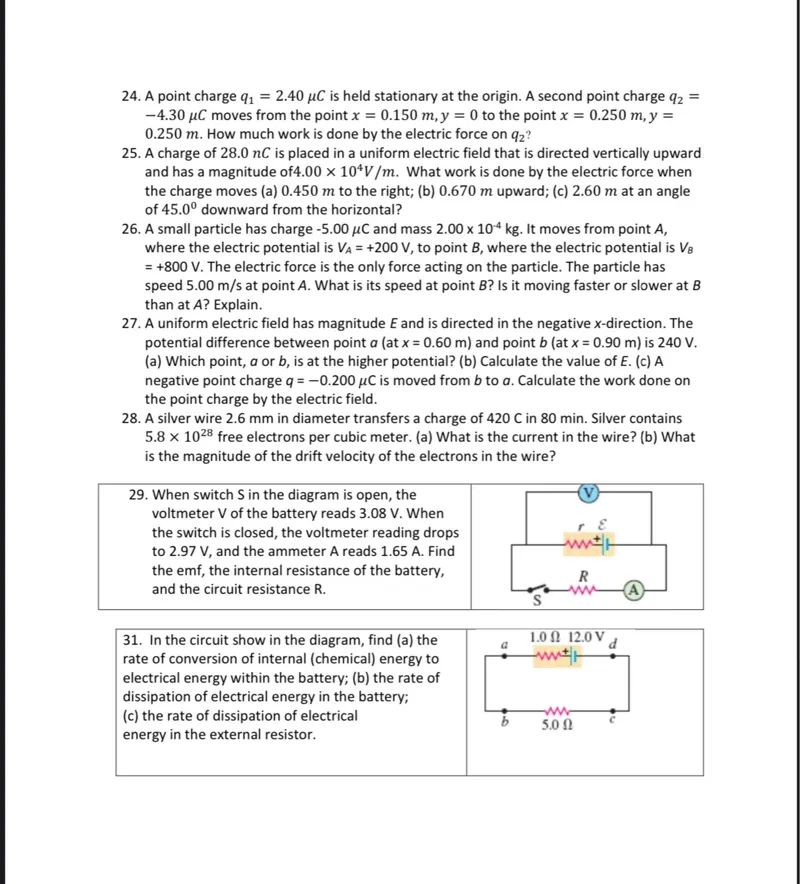
Questions: 24. A point charge q1=2.40 μC is held stationary at the origin. A second point charge q2= -4.30 μC moves from the point x=0.150 m, y=0 to the point x=0.250 m, y= 0.250 m. How much work is done by the electric force on q2? 25. A charge of 28.0 nC is placed in a uniform electric field that is directed vertically upward and has a magnitude of 4.00 x 10^4 V/m. What work is done by the electric force when the charge moves (a) 0.450 m to the right; (b) 0.670 m upward; (c) 2.60 m at an angle of 45.0° downward from the horizontal? 26. A small particle has charge -5.00 μC and mass 2.00 x 10^-4 kg. It moves from point A, where the electric potential is VA=+200 V, to point B, where the electric potential is VB =+800 V. The electric force is the only force acting on the particle. The particle has speed 5.00 m/s at point A. What is its speed at point B? Is it moving faster or slower at B than at A? Explain. 27. A uniform electric field has magnitude E and is directed in the negative x-direction. The potential difference between point a (at x=0.60 m) and point b (at x=0.90 m) is 240 V. (a) Which point, a or b, is at the higher potential? (b) Calculate the value of E. (c) A negative point charge q=-0.200 μC is moved from b to a. Calculate the work done on the point charge by the electric field. 28. A silver wire 2.6 mm in diameter transfers a charge of 420 C in 80 min. Silver contains 5.8 x 10^28 free electrons per cubic meter. (a) What is the current in the wire? (b) What is the magnitude of the drift velocity of the electrons in the wire? 29. When switch S in the diagram is open, the voltmeter V of the battery reads 3.08 V. When the switch is closed, the voltmeter reading drops to 2.97 V, and the ammeter A reads 1.65 A. Find the emf, the internal resistance of the battery, and the circuit resistance R. 31. In the circuit shown in the diagram, find (a) the rate of conversion of internal (chemical) energy to electrical energy within the battery; (b) the rate of dissipation of electrical energy in the battery; (c) the rate of dissipation of electrical energy in the external resistor.

A point charge \(q_{1}=2.40 \mu \mathrm{C}\) is held stationary at the origin. A second point charge \(q_{2}=-4.30 \mu C\) moves from the point \(x=0.150 m, y=0\) to the point \(x=0.250 m, y= 0.250 m\). How much work is done by the electric force on \(q_{2}\)?

Calculate the initial distance \(r_1\) between \(q_1\) and \(q_2\).

Initial coordinates of \(q_2\) are \((0.150, 0)\), and \(q_1\) is at the origin \((0,0)\). Thus, the initial distance is \(r_1 = \sqrt{(0.150 - 0)^2 + (0 - 0)^2} = 0.150 \ m\).

Calculate the final distance \(r_2\) between \(q_1\) and \(q_2\).

Final coordinates of \(q_2\) are \((0.250, 0.250)\), and \(q_1\) is at the origin \((0,0)\). Thus, the final distance is \(r_2 = \sqrt{(0.250 - 0)^2 + (0.250 - 0)^2} = \sqrt{0.250^2 + 0.250^2} = \sqrt{2 \cdot 0.250^2} = 0.250\sqrt{2} \approx 0.354 \ m\).

Calculate the work done by the electric force.

The work done by the electric force is given by \(W = k q_1 q_2 (1/r_1 - 1/r_2)\), where \(k = 8.988 \times 10^9 N m^2/C^2\). \(q_1 = 2.40 \times 10^{-6} C\) and \(q_2 = -4.30 \times 10^{-6} C\). \(W = (8.988 \times 10^9)(2.40 \times 10^{-6})(-4.30 \times 10^{-6})(1/0.150 - 1/0.354)\) \(W = -0.0933 \times (6.67 - 2.83) = -0.0933 \times 3.84 = -0.358 J\)

\(\boxed{-0.358 \ J}\)

A charge of 28.0 nC is placed in a uniform electric field that is directed vertically upward and has a magnitude of \(4.00 \times 10^{4} \mathrm{~V} / \mathrm{m}\). What work is done by the electric force when the charge moves (a) 0.450 m to the right; (b) 0.670 m upward; (c) 2.60 m at an angle of \(45.0^{\circ}\) downward from the horizontal?

Calculate the work done when the charge moves 0.450 m to the right (a).

Since the electric field is vertical and the displacement is horizontal, the work done is zero. \(W = qEd\cos\theta\), where \(\theta = 90^\circ\). Therefore, \(W = 0\).

Calculate the work done when the charge moves 0.670 m upward (b).

\(W = qEd\cos\theta\), where \(q = 28.0 \times 10^{-9} C\), \(E = 4.00 \times 10^4 V/m\), \(d = 0.670 m\), and \(\theta = 0^\circ\) since the displacement is in the same direction as the electric field. \(W = (28.0 \times 10^{-9})(4.00 \times 10^4)(0.670) = 7.50 \times 10^{-4} J\).

Calculate the work done when the charge moves 2.60 m at an angle of \(45.0^{\circ}\) downward from the horizontal (c).

The angle between the electric field (upward) and the displacement (45 degrees downward from the horizontal) is \(180^\circ - 45^\circ = 135^\circ\). The vertical displacement is \(d \sin(-45^\circ) = 2.60 \times \sin(-45^\circ)\) m. \(W = qEd\cos\theta = (28.0 \times 10^{-9})(4.00 \times 10^4)(2.60 \cos(135^\circ)) = -2.06 \times 10^{-3} J\).

\(\boxed{(a) \ 0 \ J, (b) \ 7.50 \times 10^{-4} \ J, (c) \ -2.06 \times 10^{-3} \ J}\)

A small particle has charge \(-5.00 \mu \mathrm{C}\) and mass \(2.00 \times 10^{-4} \mathrm{~kg}\). It moves from point \(A\), where the electric potential is \(V_{A}=+200 \mathrm{~V}\), to point \(B\), where the electric potential is \(V_{B}=+800 \mathrm{~V}\). The electric force is the only force acting on the particle. The particle has speed \(5.00 \mathrm{~m} / \mathrm{s}\) at point \(A\). What is its speed at point \(B\)? Is it moving faster or slower at \(B\) than at \(A\)? Explain.

Use conservation of energy.

\(K_A + U_A = K_B + U_B\). \(K = \frac{1}{2}mv^2\) and \(U = qV\). \(\frac{1}{2}mv_A^2 + qV_A = \frac{1}{2}mv_B^2 + qV_B\). We are given \(v_A = 5.00 \ m/s\). We want to solve for \(v_B\).

Solve for \(v_B\).

\(\frac{1}{2}(2.00 \times 10^{-4})(5.00)^2 + (-5.00 \times 10^{-6})(200) = \frac{1}{2}(2.00 \times 10^{-4})v_B^2 + (-5.00 \times 10^{-6})(800)\) \(2.5 \times 10^{-3} - 1.0 \times 10^{-3} = 1.0 \times 10^{-4}v_B^2 - 4.0 \times 10^{-3}\) \(1.5 \times 10^{-3} + 4.0 \times 10^{-3} = 1.0 \times 10^{-4}v_B^2\) \(5.5 \times 10^{-3} = 1.0 \times 10^{-4}v_B^2\) \(v_B^2 = 55\) \(v_B = \sqrt{55} = 7.42 \ m/s\) The particle is moving faster at B than at A.

\(\boxed{7.42 \ m/s}\) The particle is moving faster at B.

\(-0.358 \ J\) \((a) \ 0 \ J, (b) \ 7.50 \times 10^{-4} \ J, (c) \ -2.06 \times 10^{-3} \ J\) \(7.42 \ m/s\) The particle is moving faster at B.