Questions: a) Find the derivative of the function g̅(x)=4x b) Evaluate g′(3) c) Find the equation of the line tangent to g(x)=4x where x=1 d) Find the point on the graph of g(x)=√(-4)x where the tangent line to the curve will be parallel to the line y=2x-3

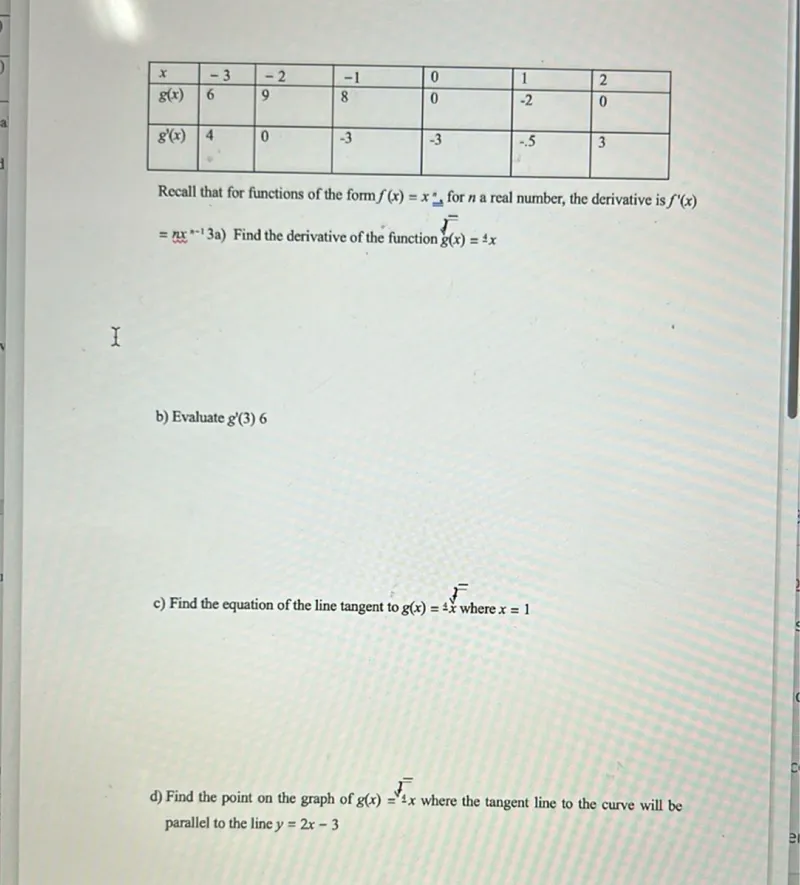
a) Find the derivative of the function \(\bar{g}(x) = 4x\)

Derivative of a linear function

The derivative of a function of the form \(f(x) = mx\) is \(f'(x) = m\). Here, \(\bar{g}(x) = 4x\), so the derivative is \(\bar{g}'(x) = 4\).

\(\boxed{\bar{g}'(x) = 4}\)

b) Evaluate \(g'(3)\)

Check the table for \(g'(3)\)

The table provides values of \(g'(x)\) for \(x = -3, -2, -1, 0, 1, 2\). Since \(x = 3\) is not in the table, \(g'(3)\) cannot be determined from the given information.

\(\boxed{\text{Not determinable from the table}}\)

c) Find the equation of the line tangent to \(g(x) = 4x\) where \(x = 1\)

Find the slope of the tangent line

The derivative of \(g(x) = 4x\) is \(g'(x) = 4\). At \(x = 1\), the slope of the tangent line is \(g'(1) = 4\).

Find the point on the curve at \(x = 1\)

At \(x = 1\), \(g(1) = 4(1) = 4\). The point is \((1, 4)\).

Write the equation of the tangent line

Using the point-slope form \(y - y_1 = m(x - x_1)\), the equation is \(y - 4 = 4(x - 1)\), which simplifies to \(y = 4x\).

\(\boxed{y = 4x}\)

d) Find the point on the graph of \(g(x) = \sqrt{-4}x\) where the tangent line to the curve will be parallel to the line \(y = 2x - 3\)

Analyze the function \(g(x) = \sqrt{-4}x\)

The expression \(\sqrt{-4}\) is not a real number, so the function \(g(x) = \sqrt{-4}x\) is not defined for real \(x\).

\(\boxed{\text{The function is not defined for real numbers}}\)

a) \(\boxed{\bar{g}'(x) = 4}\)
b) \(\boxed{\text{Not determinable from the table}}\)
c) \(\boxed{y = 4x}\)
d) \(\boxed{\text{The function is not defined for real numbers}}\)