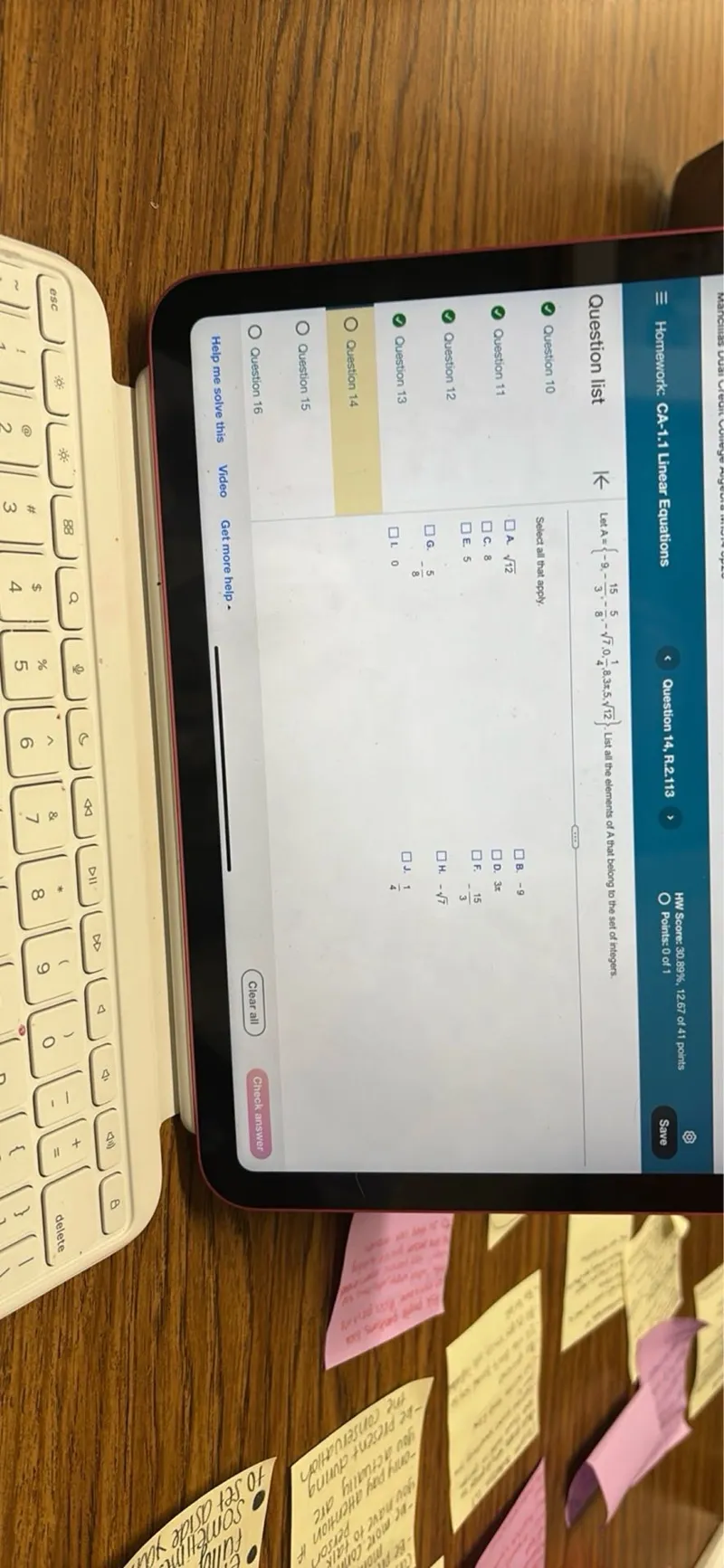
Questions: Homework: Ch4-11 Linear Equations Last test : 9:15 (2x + 3)(x^2 - 7) Last answer of A: Teacher is here. A. x^3 - 7x + 3x^2 - 21 B. 2x^3 - 14x + 3x^2 - 21 C. 2x^3 + 3x^2 - 14x - 21 D. 2x^3 - 14x + 3x^2 - 21 E. None of the above

Solution Steps

The given expression is \((2x + 3)(x^2 - 7)\). To expand this, we use the distributive property (also known as the FOIL method for binomials):

\[ (2x + 3)(x^2 - 7) = 2x \cdot x^2 + 2x \cdot (-7) + 3 \cdot x^2 + 3 \cdot (-7) \]

Now, simplify each term in the expansion:

\[ 2x \cdot x^2 = 2x^3 \] \[ 2x \cdot (-7) = -14x \] \[ 3 \cdot x^2 = 3x^2 \] \[ 3 \cdot (-7) = -21 \]

Combine all the simplified terms:

\[ 2x^3 - 14x + 3x^2 - 21 \]

Now, compare the expanded expression with the provided options:

• A. \(x^3 - 7x + 3x^2 - 21\)
• B. \(2x^3 - 14x + 3x^2 - 21\)
• C. \(2x^3 + 3x^2 - 14x - 21\)
• D. \(2x^3 - 14x + 3x^2 - 21\)
• E. None of the above

The expanded expression matches B and D. However, since both B and D are identical, the correct answer is either B or D.

Final Answer