Questions: Find the domain and range of the relation, and then determine whether the relation represents a function. The domain for the given relation is β. (Use a comma to separate answers as needed.)

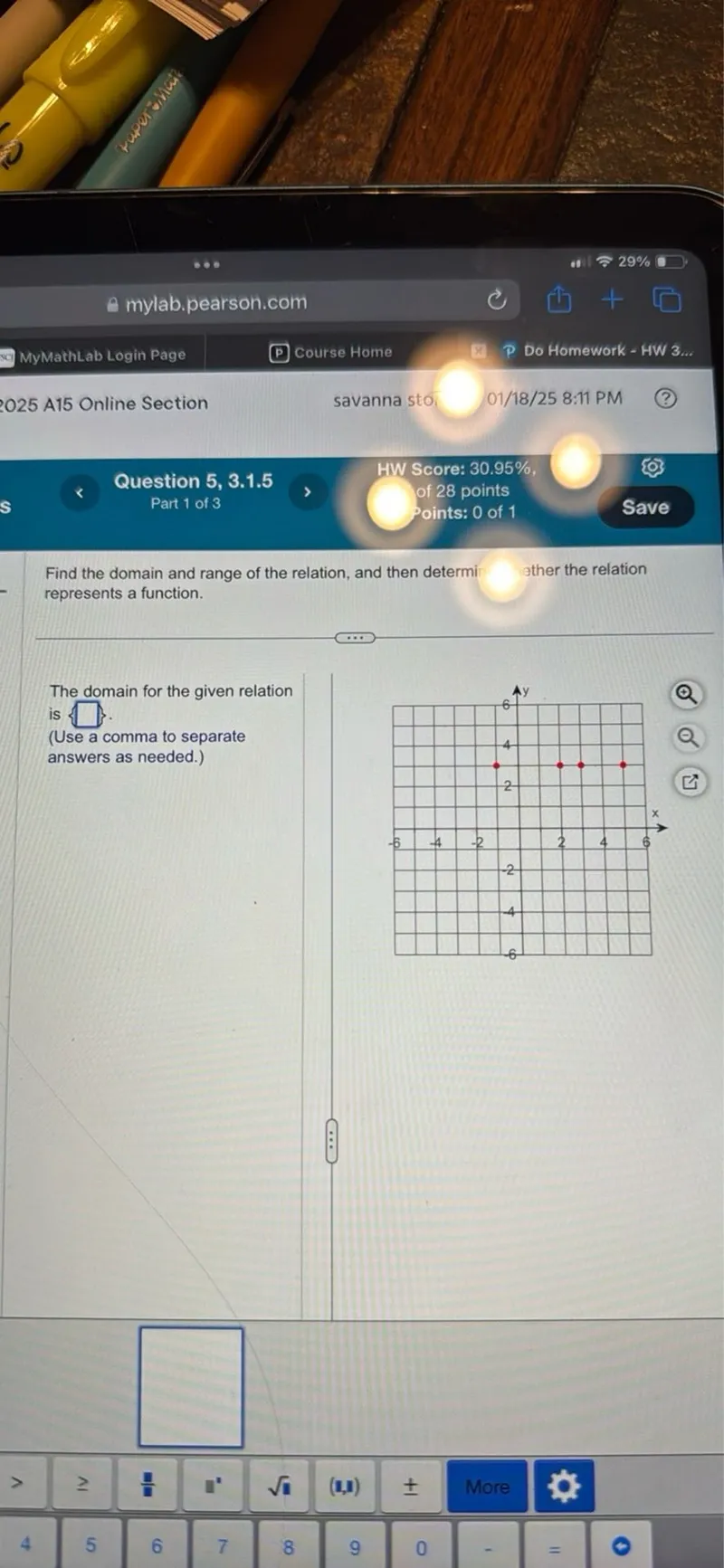
Find the domain and range of the relation, and then determine whether the relation represents a function.

The domain for the given relation is β. (Use a comma to separate answers as needed.)

Transcript text: Find the domain and range of the relation, and then determine whether the relation represents a function. The domain for the given relation is $\square$ $\beta$. (Use a comma to separate answers as needed.)

Solution

Solution Steps

Step 1: Identify the points in the graph

The points in the graph are (-1, 3), (2, 3), (3, 3), and (5, 3).

Step 2: Find the domain

The domain of a relation is the set of all possible x-values. In this case, the x-values are -1, 2, 3, and 5.

Step 3: Find the range

The range of a relation is the set of all possible y-values. In this case, the y-value is 3 for all points.

Step 4: Determine if the relation is a function

A relation is a function if each x-value corresponds to only one y-value. In this case, each x-value has a unique y-value. Therefore, the relation is a function.

Final Answer

Domain: $-1, 2, 3, 5$ Range: $3$ Is it a function?: Yes

Was this solution helpful?

Unhelpful

Helpful

Questions asked by other users

< Previous Next >

Thank you for your feedback.

Copied!