Questions: 1. Assume Hudson has a target income of 150,000. What amount of sales (in dollars) is needed to produce this target income? 2. If Hudson achieves its target income, what is its margin of safety (in percent)?
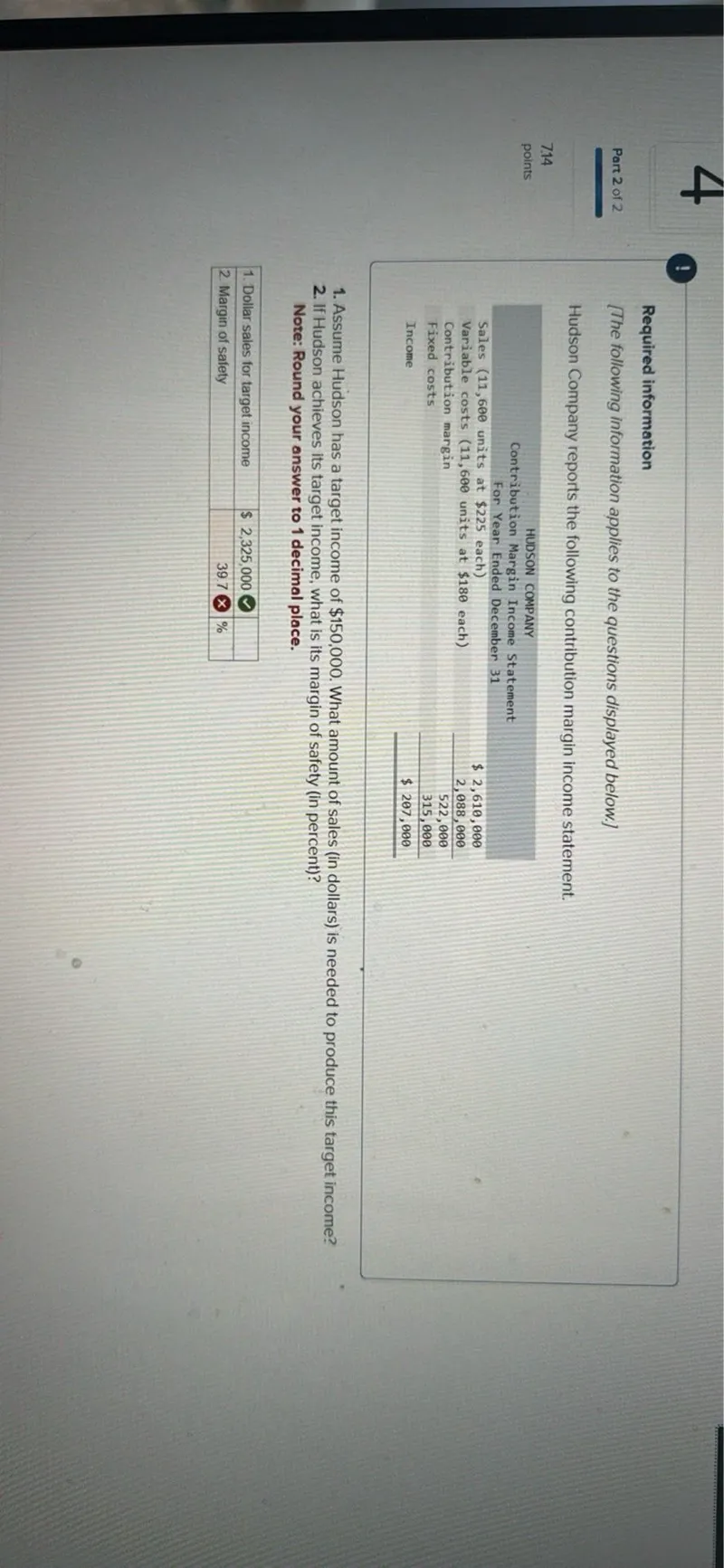
Transcript text: 1. Assume Hudson has a target income of $\$ 150,000$. What amount of sales (in dollars) is needed to produce this target income?
2. If Hudson achieves its target income, what is its margin of safety (in percent)?





