Questions: Determine the rate of reaction for the following chemical reaction, based on the information given: 2 H2O2(aq) → 2 H2O(l) + O2(g) Reaction started with 1.4 M H2O2 and ended with 0.6 M after 0.8 minutes. Make your answer's precision to two decimal places. Include the correct units in your answer for full credit.

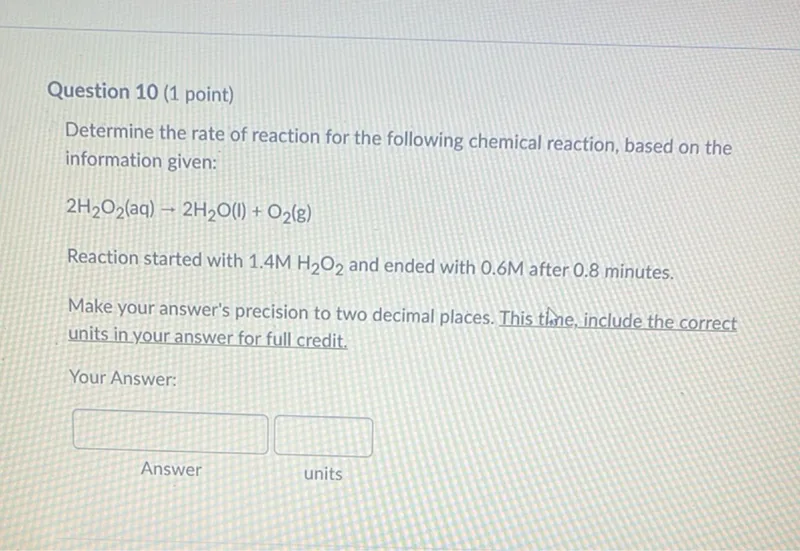
Determine the rate of reaction for the given chemical reaction.

Calculate the change in concentration of \( \mathrm{H}_{2} \mathrm{O}_{2} \).

The initial concentration of \( \mathrm{H}_{2} \mathrm{O}_{2} \) is \(1.4 \, \mathrm{M}\), and the final concentration is \(0.6 \, \mathrm{M}\). The change in concentration is given by:
\[ \Delta [\mathrm{H}_{2} \mathrm{O}_{2}] = [\mathrm{H}_{2} \mathrm{O}_{2}]_{\text{final}} - [\mathrm{H}_{2} \mathrm{O}_{2}]_{\text{initial}} = 0.6 \, \mathrm{M} - 1.4 \, \mathrm{M} = -0.8 \, \mathrm{M} \]

Calculate the rate of reaction.

The rate of reaction is defined as the change in concentration of a reactant or product per unit time. For the decomposition of \( \mathrm{H}_{2} \mathrm{O}_{2} \), the rate is:
\[ \text{Rate} = -\frac{\Delta [\mathrm{H}_{2} \mathrm{O}_{2}]}{\Delta t} = -\frac{-0.8 \, \mathrm{M}}{0.8 \, \text{minutes}} = 1.0 \, \mathrm{M/min} \]
The negative sign indicates the concentration of \( \mathrm{H}_{2} \mathrm{O}_{2} \) is decreasing, but the rate is expressed as a positive value.

\(\boxed{1.0 \, \mathrm{M/min}}\)

\(\boxed{1.0 \, \mathrm{M/min}}\)