Questions: For the following set of data, find the sample standard deviation, to the nearest hundredth. Data Frequency --- --- 2 3 3 8 6 2 8 4 16 6 17 2 19 1 20 9
Transcript text: Solution
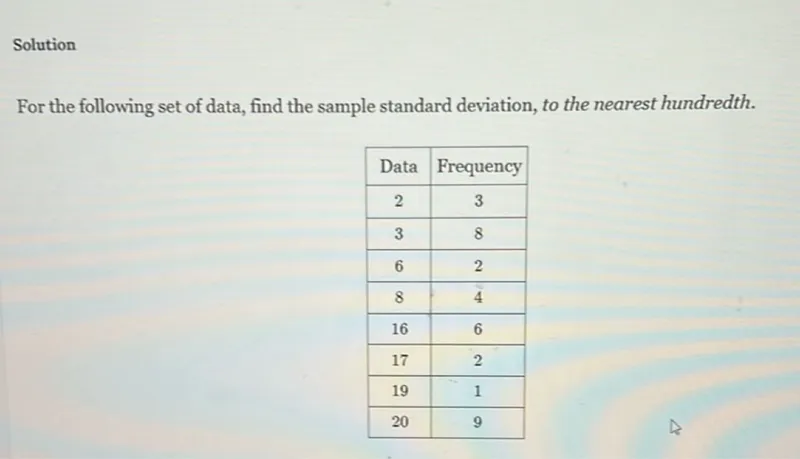
For the following set of data, find the sample standard deviation, to the nearest hundredth.
\begin{tabular}{|c|c|}
\hline Data & Frequency \\
\hline 2 & 3 \\
\hline 3 & 8 \\
\hline 6 & 2 \\
\hline 8 & 4 \\
\hline 16 & 6 \\
\hline 17 & 2 \\
\hline 19 & 1 \\
\hline 20 & 9 \\
\hline
\end{tabular}





