Questions: Select the correct answer. What are the solutions of the quadratic equation below? 2 x^2 - 2 x - 9 = 0 A. (-1 ± √19)/2 B. (3 ± √19)/2 C. (1 ± √19)/2 D. (1 ± 3√19)/2
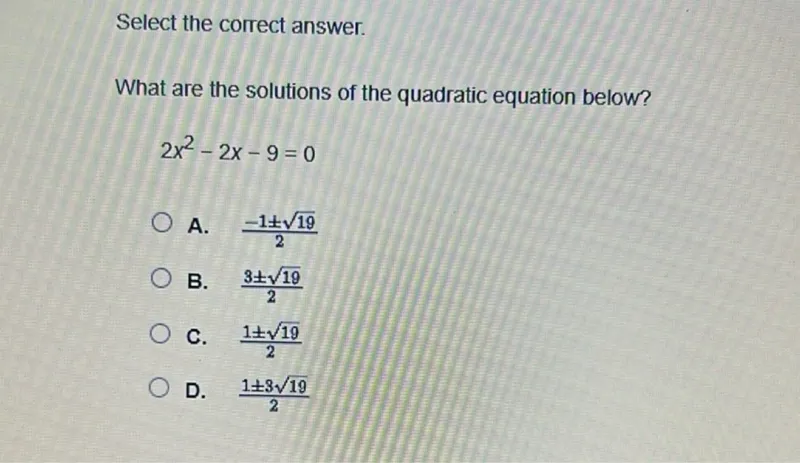
Transcript text: Select the correct answer.
What are the solutions of the quadratic equation below?
\[
2 x^{2}-2 x-9=0
\]
A. $\frac{-1 \pm \sqrt{19}}{2}$
B. $\frac{3 \pm \sqrt{19}}{2}$
C. $\frac{1 \pm \sqrt{19}}{2}$
D. $\frac{1 \pm 3 \sqrt{19}}{2}$





