Questions: The figure below was made with a scale of 1 unit = 3 m. Draw the figure with a new scale of 1 unit = 9 m. You can place your figure anywhere on the grid on the right.

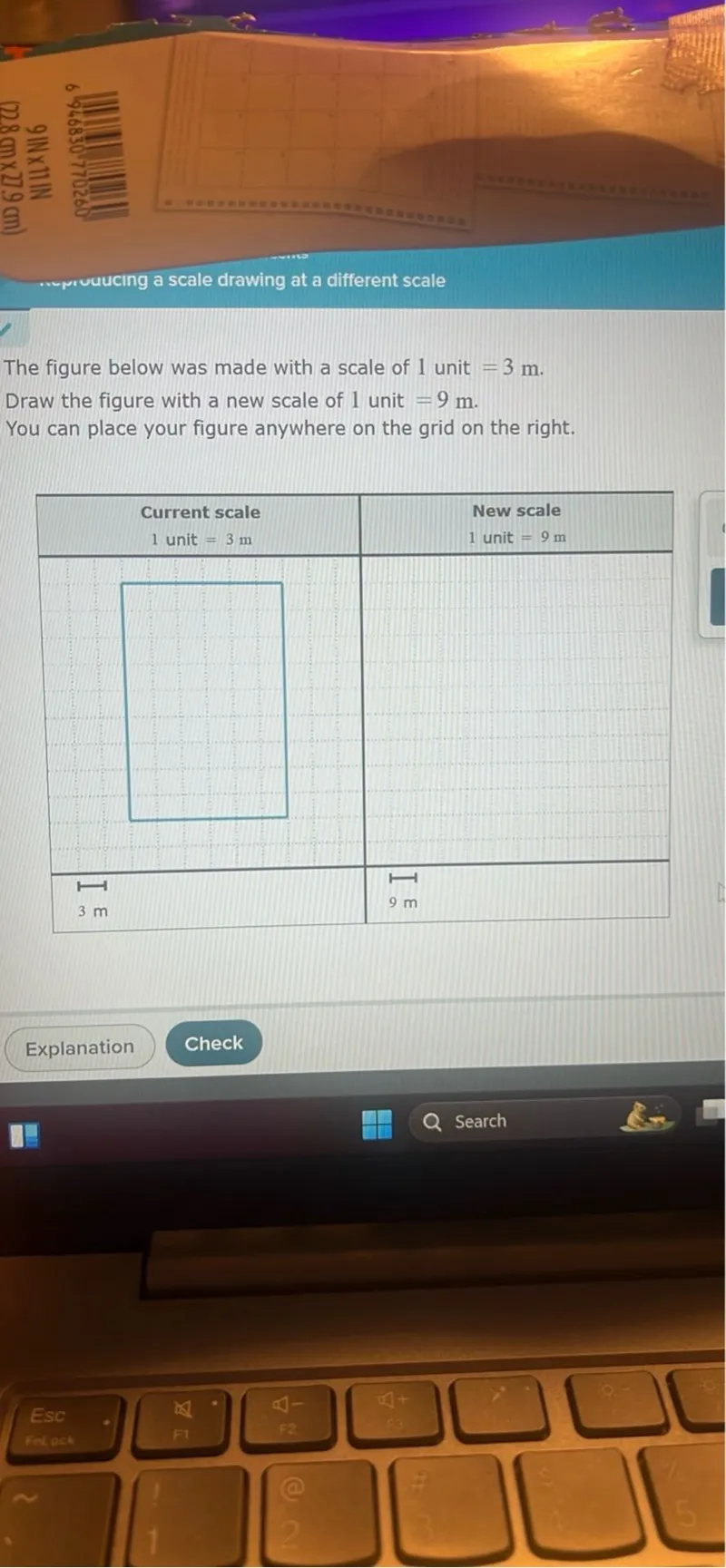
The figure below was made with a scale of 1 unit = 3 m. Draw the figure with a new scale of 1 unit = 9 m. You can place your figure anywhere on the grid on the right.

Transcript text: The figure below was made with a scale of 1 unit $=3 \mathrm{~m}$. Draw the figure with a new scale of 1 unit $=9 \mathrm{~m}$. You can place your figure anywhere on the grid on the right.

Solution

Solution Steps

Step 1: Understanding the Scale Change

The current scale is 1 unit = 3 meters. The new scale is 1 unit = 9 meters. This means that each unit in the new scale represents three times the distance of the current scale.

Step 2: Calculating the Scale Factor

To convert the figure from the current scale to the new scale, we need to determine the scale factor. The scale factor is the ratio of the new scale to the current scale: \[ \text{Scale Factor} = \frac{9 \text{ meters/unit}}{3 \text{ meters/unit}} = 3 \]

Step 3: Applying the Scale Factor

To draw the figure with the new scale, each dimension of the figure should be divided by the scale factor of 3. This will reduce the size of the figure to fit the new scale.