Questions: Solve the compound inequality. Graph the solution set, and write the solution set in interval notation if possible. Write the numbers as simplified fractions or integers. (a) - 8 ≤ 3 or 5 ≥ 2 (b) - 7 ≤ 3 and 5 ≤ 2

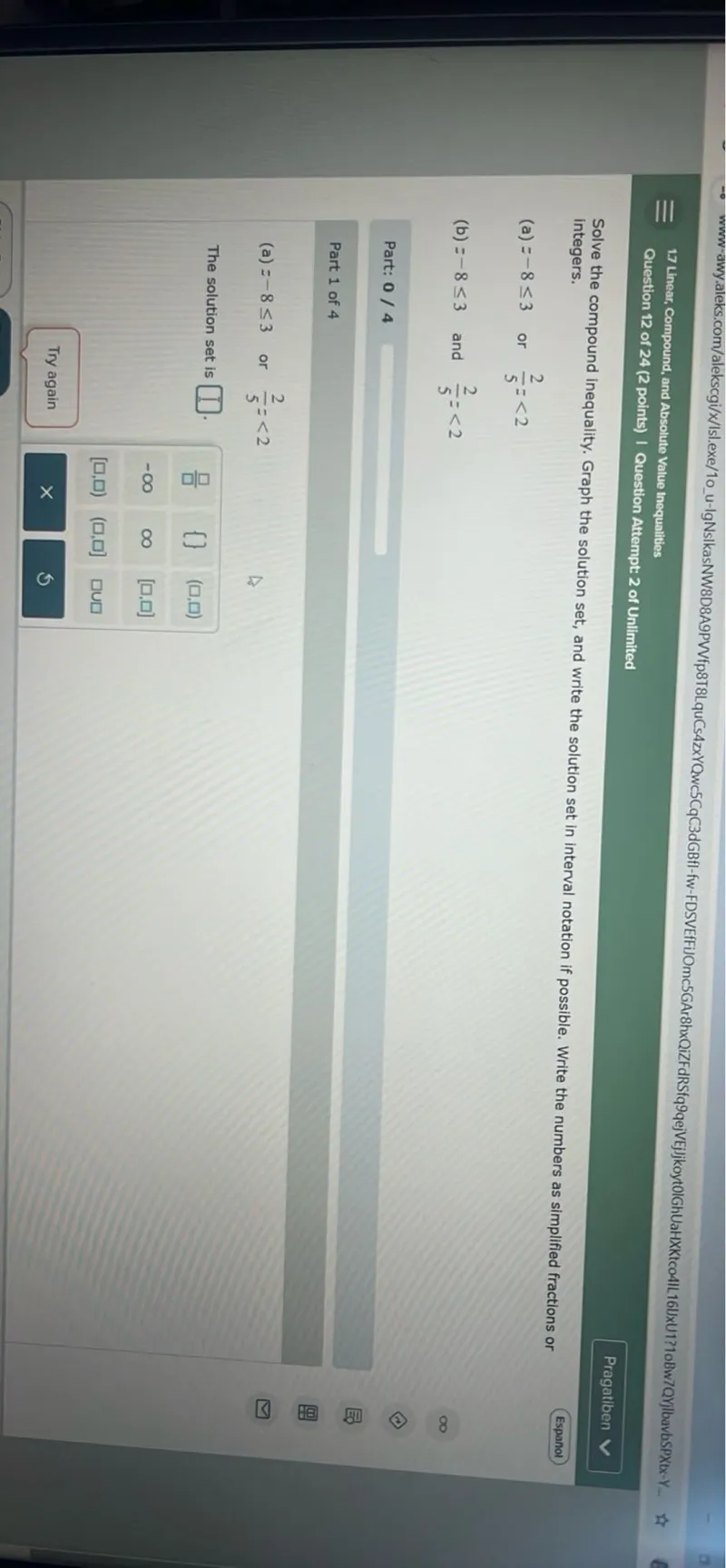
Solve the compound inequality (a) \(-8 \leq 3\) or \(5 \geq 2\).

Evaluate the first inequality \(-8 \leq 3\).

The inequality \(-8 \leq 3\) is true because \(-8\) is indeed less than or equal to \(3\).

Evaluate the second inequality \(5 \geq 2\).

The inequality \(5 \geq 2\) is true because \(5\) is indeed greater than or equal to \(2\).

Determine the solution set for the compound inequality using "or".

Since both inequalities are true, the solution set for the compound inequality is all real numbers.

\(\boxed{(-\infty, \infty)}\)

Solve the compound inequality (b) \(-7 \leq 3\) and \(5 \leq 2\).

Evaluate the first inequality \(-7 \leq 3\).

The inequality \(-7 \leq 3\) is true because \(-7\) is indeed less than or equal to \(3\).

Evaluate the second inequality \(5 \leq 2\).

The inequality \(5 \leq 2\) is false because \(5\) is not less than or equal to \(2\).

Determine the solution set for the compound inequality using "and".

Since the second inequality is false, the solution set for the compound inequality is the empty set.

\(\boxed{\emptyset}\)

\((- \infty, \infty)\)
\(\emptyset\)