Questions: Attempt 1:5 attempts remaining. Consider the function, g(x)=x^2-2x. Complete the table below, then estimate the instantaneous rate of change of the function g at x=4. Fixed Endpoint Other Endpoint Δx Slope of Secant Line (Average Rate of Change) ------------ (4,8) (3.9,7.41) 0.1 5.9 (4,8) (3.99,7.9401) 0.01 (4,8) (3.999,7.994) 0.001 5.999 (4,8) (3.9999,7.9994) 0.0001 (4,8) (4.1,8.61) -0.1 6.1 (4,8) (4.01,8.0601) -0.01 6.01 (4,8) (4.001,8.006) -0.001 (4,8) (4.0001,8.0006) -0.0001 Estimate of the instantaneous rate of change of the function g at x=4 :

Attempt 1:5 attempts remaining.

Consider the function, g(x)=x^2-2x. Complete the table below, then estimate the instantaneous rate of change of the function g at x=4.

Fixed Endpoint Other Endpoint Δx Slope of Secant Line (Average Rate of Change)
------------
(4,8) (3.9,7.41) 0.1 5.9
(4,8) (3.99,7.9401) 0.01
(4,8) (3.999,7.994) 0.001 5.999
(4,8) (3.9999,7.9994) 0.0001
(4,8) (4.1,8.61) -0.1 6.1
(4,8) (4.01,8.0601) -0.01 6.01
(4,8) (4.001,8.006) -0.001
(4,8) (4.0001,8.0006) -0.0001

Estimate of the instantaneous rate of change of the function g at x=4 :

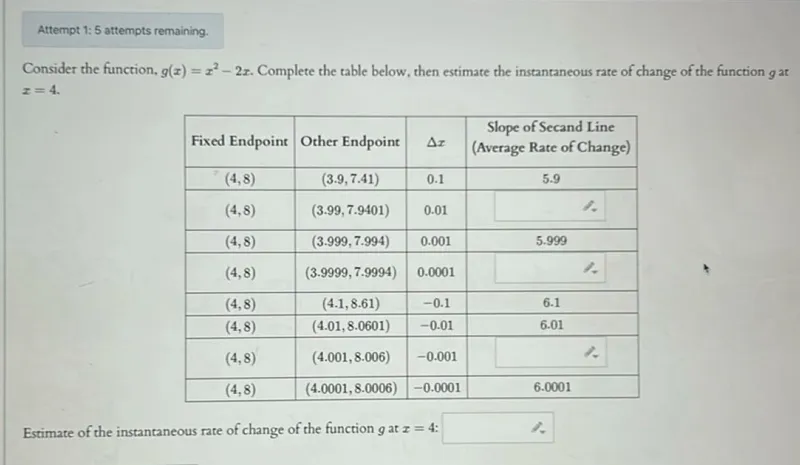
Transcript text: Attempt 1:5 attempts remaining. Consider the function, $g(x)=x^{2}-2 x$. Complete the table below, then estimate the instantaneous rate of change of the function $g$ at $x=4$. \begin{tabular}{|c|c|c|c|} \hline Fixed Endpoint & Other Endpoint & $\Delta x$ & \begin{tabular}{c} Slope of Secand Line \\ (Average Rate of Change) \end{tabular} \\ \hline$(4,8)$ & $(3.9,7.41)$ & 0.1 & 5.9 \\ \hline$(4,8)$ & $(3.99,7.9401)$ & 0.01 & \\ \hline$(4,8)$ & $(3.999,7.994)$ & 0.001 & 5.999 \\ \hline$(4,8)$ & $(3.9999,7.9994)$ & 0.0001 & \\ \hline$(4,8)$ & $(4.1,8.61)$ & -0.1 & 6.1 \\ \hline$(4,8)$ & $(4.01,8.0601)$ & -0.01 & 6.01 \\ \hline$(4,8)$ & $(4.001,8.006)$ & -0.001 & \\ \hline$(4,8)$ & $(4.0001,8.0006)$ & -0.0001 & \\ \hline \end{tabular} Estimate of the instantaneous rate of change of the function $g$ at $x=4$ :

Solution

Solution Steps

Step 1: Calculate the Average Rate of Change for $ \Delta x = 0.01 $

To find the average rate of change of the function $ g(x) = x^2 - 2x $ between the points $ (4, 8) $ and $ (3.99, 7.9401) $, we compute:

\[ \text{Average Rate of Change} = \frac{g(3.99) - g(4)}{3.99 - 4} = \frac{7.9401 - 8}{3.99 - 4} = 5.99 \]

Step 2: Calculate the Average Rate of Change for $ \Delta x = 0.0001 $

Next, we calculate the average rate of change between the points $ (4, 8) $ and $ (4.0001, 8.0006) $:

\[ \text{Average Rate of Change} = \frac{g(4.0001) - g(4)}{4.0001 - 4} = \frac{8.0006 - 8}{4.0001 - 4} = 6.0001 \]

Step 3: Calculate the Average Rate of Change for $ \Delta x = -0.001 $

We then find the average rate of change between the points $ (4, 8) $ and $ (4.001, 8.006) $:

\[ \text{Average Rate of Change} = \frac{g(4.001) - g(4)}{4.001 - 4} = \frac{8.006 - 8}{4.001 - 4} = 6.001 \]

Step 4: Calculate the Average Rate of Change for $ \Delta x = -0.0001 $

Finally, we calculate the average rate of change between the points $ (4, 8) $ and $ (4.0001, 8.0006) $:

\[ \text{Average Rate of Change} = \frac{g(4.0001) - g(4)}{4.0001 - 4} = \frac{8.0006 - 8}{4.0001 - 4} = 6.0001 \]

Step 5: Estimate the Instantaneous Rate of Change

The instantaneous rate of change of the function $ g $ at $ x = 4 $ can be estimated by observing the average rates of change as $ \Delta x $ approaches $ 0 $. The values calculated suggest that:

\[ \text{Instantaneous Rate of Change} \approx 6 \]

This value represents the slope of the tangent line to the curve at the point $ (4, 8) $.