Questions: Week 2: Ch 11 (Part 1) Solving a Systems Classifying systems of linear equations System A Line 1: y=-x-2 Line 2: y=-x-3 This system of equations is: consistent dependent consistent independent inconsistent This means the system has: a unique solution

Week 2: Ch 11 (Part 1) Solving a Systems Classifying systems of linear equations

System A

Line 1: y=-x-2

Line 2: y=-x-3

This system of equations is: consistent dependent consistent independent inconsistent

This means the system has: a unique solution

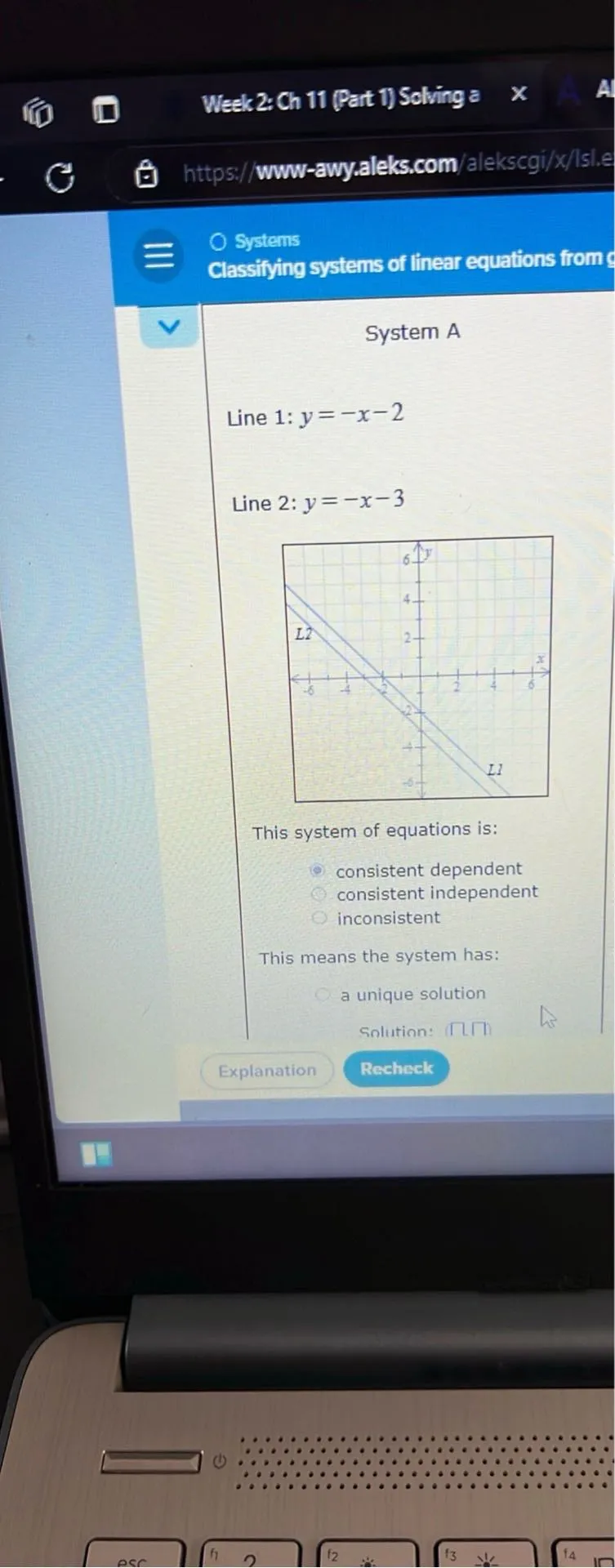
Transcript text: Week 2: Ch 11 (Part 1) Solving a Systems Classifying systems of linear equations System A Line 1: $y=-x-2$ Line 2: $y=-x-3$ This system of equations is: consistent dependent consistent independent inconsistent This means the system has: a unique solution

Solution

Solution Steps

Step 1: Analyze the slopes and y-intercepts

The given equations are: Line 1: $y = -x - 2$ Line 2: $y = -x - 3$

Both lines have the same slope, which is -1. However, they have different y-intercepts: -2 for Line 1 and -3 for Line 2.

Step 2: Determine the type of system

Since the lines have the same slope but different y-intercepts, they are parallel and will never intersect. This means there is no solution. Therefore, the system of equations is inconsistent.

Step 3: Determine the number of solutions

An inconsistent system has no solution.