Questions: Add or subtract as indicated. Express the answer as a single polynomial in standard form. 10) (2 x^2-8 x+8)+(7 x^2-4 x-2) A) 9 x^2-12 x-6 B) 15 x^2-2 x-10 C) 9 x^2+12 x+6 D) 9 x^2-12 x+6 11) (6 x^2-12 x+20)-(3 x^2-2 x-18) A) 3 x^2-10 x-38 B) 3 x^2-10 x+2 C) 3 x^2-9 x+2 D) 3 x^2-10 x+38 12) (-10 x^2+5)-(-x^3+2 x^2+1) A) x^3-8 x^2+6 B) -9 x^3+7 x^2-1 C) x^3-12 x^2+4 D) -9 x^3+2 x^2+4 13) (3 x^7+5 x^6+6 x)+(5 x^7-8 x^6+9 x) A) 8 x^7-3 x^6+15 x B) 20 x^14 C) 8 x-3 x^7+15 x^6 D) 11 x^7-5 x^6+14 x Perform the indicated operations. Express the answer as a single polynomial in standard form. 14) 3 x^5(-5 x^6-1) A) -15 x^6-3 B) -18 x^5 C) -15 x^11-3 x^5 D) -15 x^11-1 Multiply the polynomials using the FOIL method. Express the answer as a single polynomial in standard form. 15) (-4 x+5)(x+11) A) -4 x^2-39 x-39 B) -4 x^2-41 x+55 C) -4 x^2+55 x-39 D) -4 x^2-39 x+55 16) (5 x+6)(4 x+4) A) 9 x^2+44 x+24 B) 20 x^2+44 x+44 C) 20 x^2+44 x+24 D) 9 x^2+44 x+44
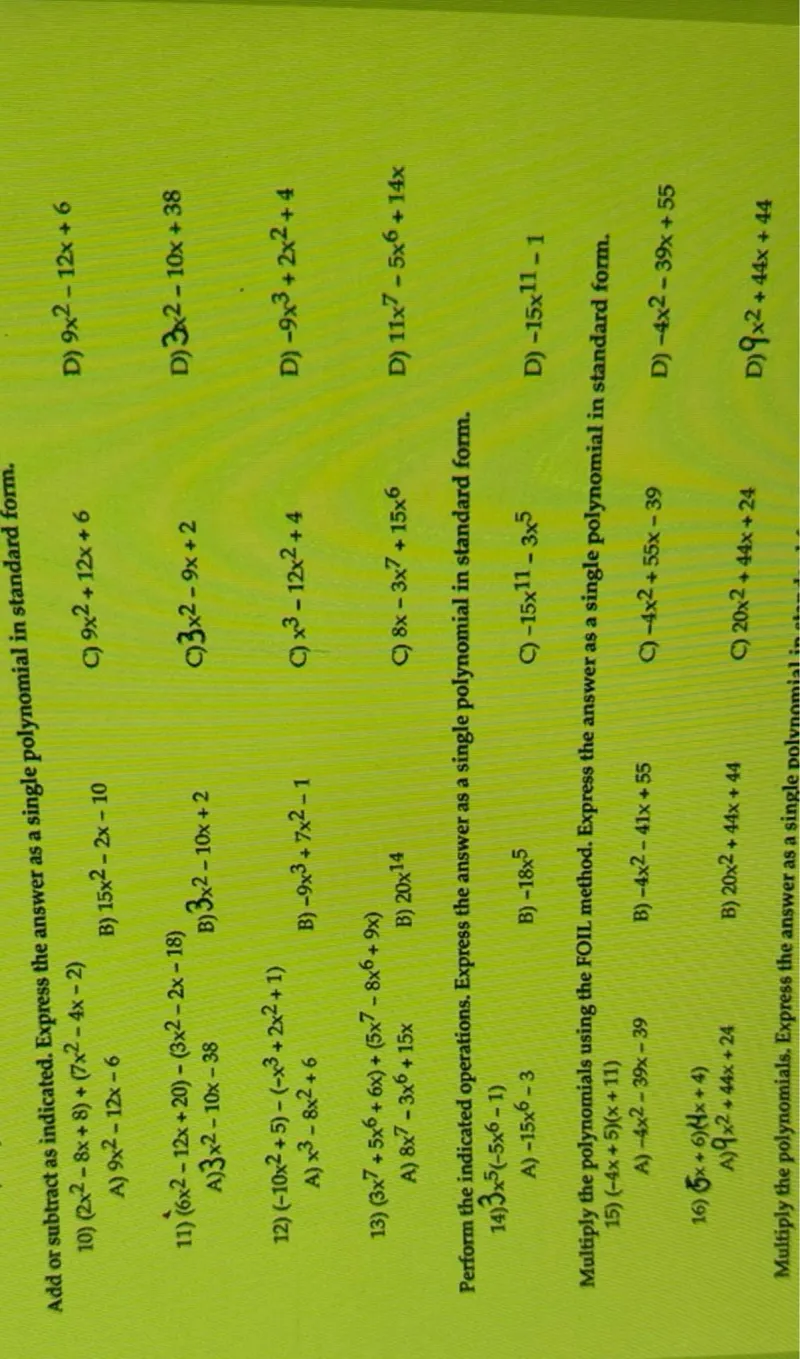
Transcript text: Add or subtract as indicated. Express the answer as a single polynomial in standard form.
10) $\left(2 x^{2}-8 x+8\right)+\left(7 x^{2}-4 x-2\right)$
A) $9 x^{2}-12 x-6$
B) $15 x^{2}-2 x-10$
C) $9 x^{2}+12 x+6$
D) $9 x^{2}-12 x+6$
11) $\left(6 x^{2}-12 x+20\right)-\left(3 x^{2}-2 x-18\right)$
A) $3 x^{2}-10 x-38$
B) $3 x^{2}-10 x+2$
C) $3 x^{2}-9 x+2$
D) $3 x^{2}-10 x+38$
12) $\left(-10 x^{2}+5\right)-\left(-x^{3}+2 x^{2}+1\right)$
A) $x^{3}-8 x^{2}+6$
B) $-9 x^{3}+7 x^{2}-1$
C) $x^{3}-12 x^{2}+4$
D) $-9 x^{3}+2 x^{2}+4$
13) $\left(3 x^{7}+5 x^{6}+6 x\right)+\left(5 x^{7}-8 x^{6}+9 x\right)$
A) $8 x^{7}-3 x^{6}+15 x$
B) $20 x^{14}$
C) $8 x-3 x^{7}+15 x^{6}$
D) $11 x^{7}-5 x^{6}+14 x$
Perform the indicated operations. Express the answer as a single polynomial in standard form.
14) $3 x^{5}\left(-5 x^{6}-1\right)$
A) $-15 x^{6}-3$
B) $-18 x^{5}$
C) $-15 x^{11}-3 x^{5}$
D) $-15 x^{11}-1$
Multiply the polynomials using the FOIL method. Express the answer as a single polynomial in standard form.
15) $(-4 x+5)(x+11)$
A) $-4 x^{2}-39 x-39$
B) $-4 x^{2}-41 x+55$
C) $-4 x^{2}+55 x-39$
D) $-4 x^{2}-39 x+55$
16) $(5 x+6)(4 x+4)$
A) $9 x^{2}+44 x+24$
B) $20 x^{2}+44 x+44$
C) $20 x^{2}+44 x+24$
D) $9 x^{2}+44 x+44$





