Questions: Find the exact value of cos^(-1)(-(sqrt(3)/2)). cos^(-1)(-(sqrt(3)/2))=-(pi/3) cos^(-1)(-(sqrt(3)/2))=(5pi/6) cos^(-1)(-(sqrt(3)/2))=-(pi/6) cos^(-1)(-(sqrt(3)/2))=(2pi/3)

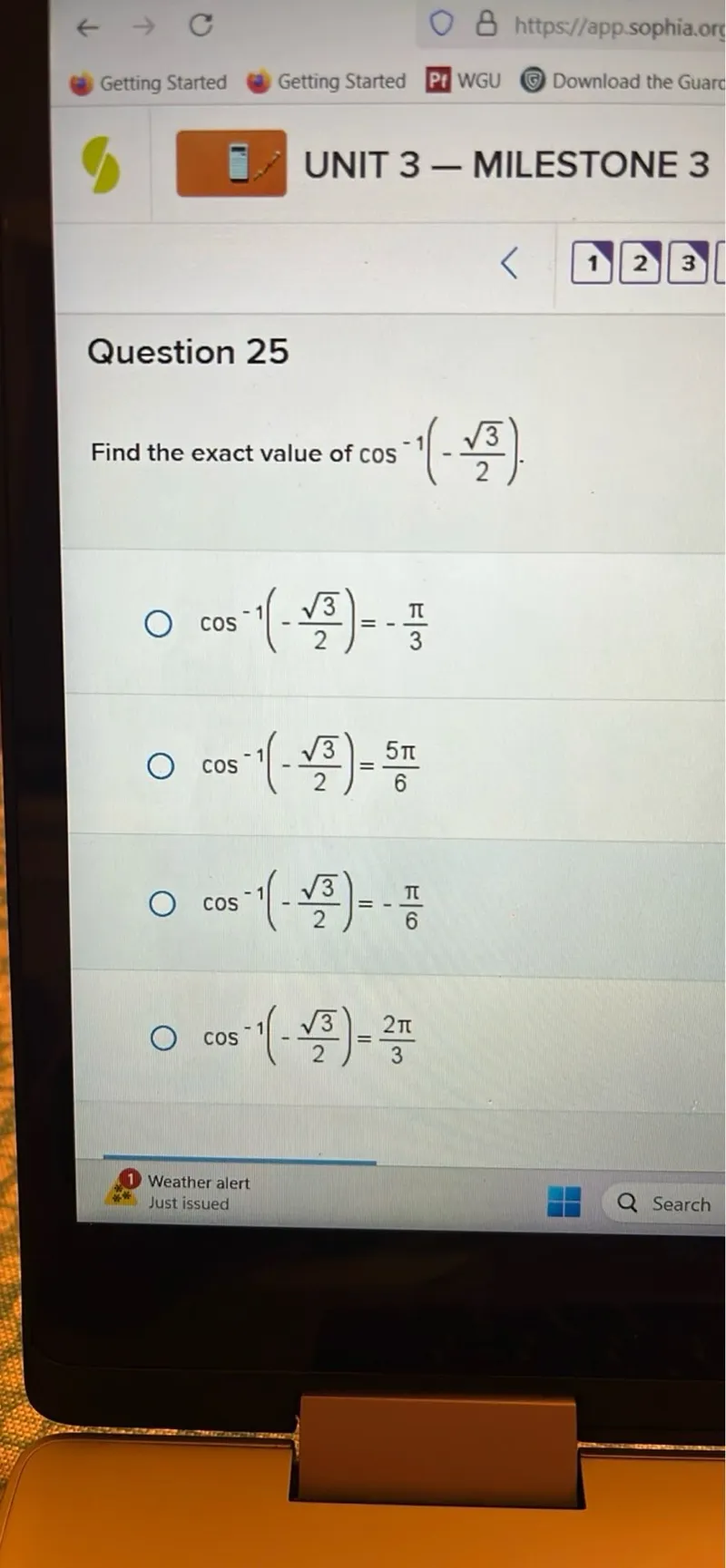
Find the exact value of \( \cos^{-1}\left(-\frac{\sqrt{3}}{2}\right) \).

Understanding the problem

We need to find the angle \( \theta \) such that \( \cos(\theta) = -\frac{\sqrt{3}}{2} \). The range of \( \cos^{-1} \) is \( [0, \pi] \), so the angle must lie in this interval.

Recall the unit circle values

We know that \( \cos\left(\frac{\pi}{6}\right) = \frac{\sqrt{3}}{2} \). Since cosine is negative in the second quadrant, the angle corresponding to \( \cos^{-1}\left(-\frac{\sqrt{3}}{2}\right) \) is \( \pi - \frac{\pi}{6} = \frac{5\pi}{6} \).

Verify the options

The options provided are:

1. \( -\frac{\pi}{3} \)
2. \( \frac{5\pi}{6} \)
3. \( -\frac{\pi}{6} \)
4. \( \frac{2\pi}{3} \)

From our calculation, the correct value is \( \frac{5\pi}{6} \).

The exact value of \( \cos^{-1}\left(-\frac{\sqrt{3}}{2}\right) \) is \( \boxed{\frac{5\pi}{6}} \).

The exact value of \( \cos^{-1}\left(-\frac{\sqrt{3}}{2}\right) \) is \( \boxed{\frac{5\pi}{6}} \).