Questions: If θ = 1π/6, then find exact values for the following: sec (θ) equals □ csc (θ) equals □ tan (θ) equals □ cot (θ) equals □
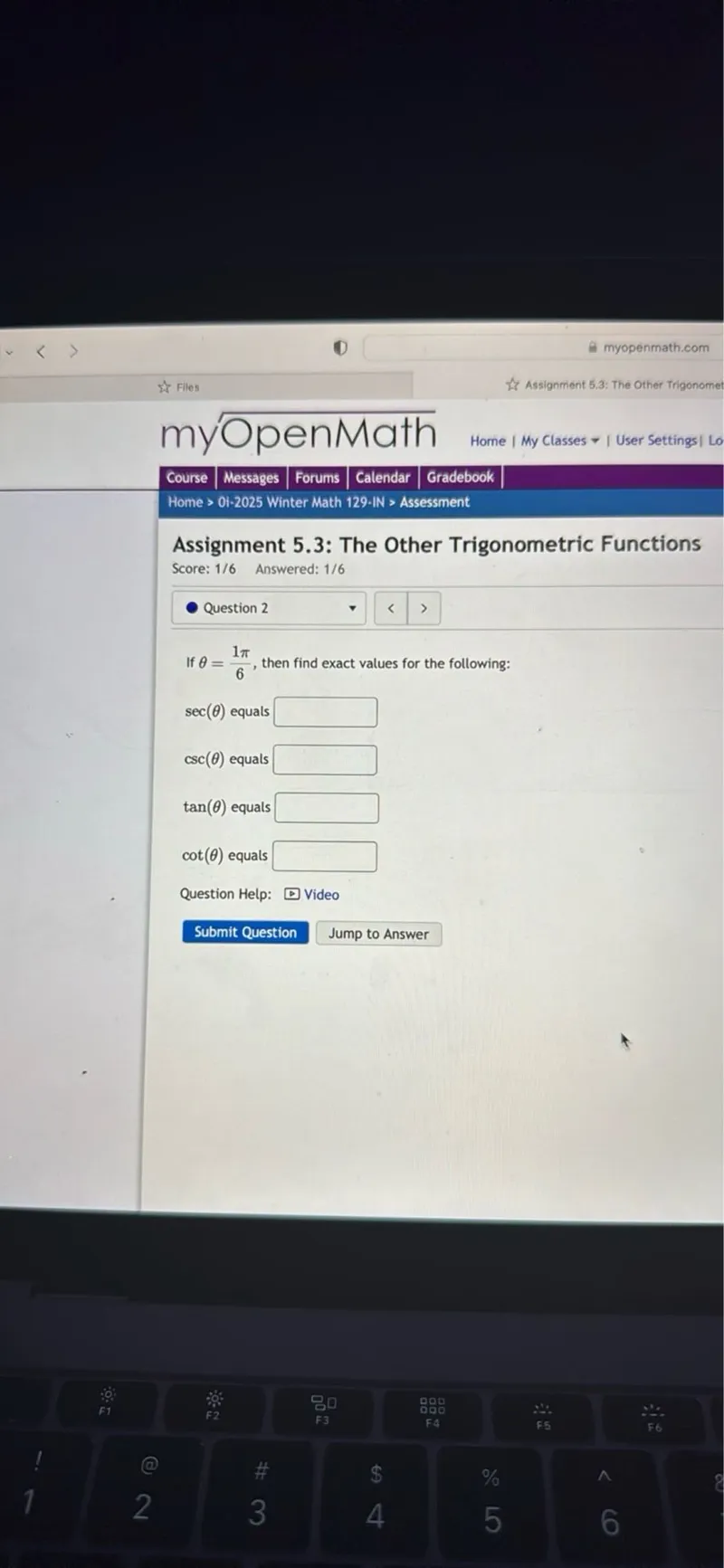
Transcript text: If $\theta=\frac{1 \pi}{6}$, then find exact values for the following:
$\sec (\theta)$ equals $\square$
$\csc (\theta)$ equals $\square$
$\tan (\theta)$ equals $\square$
$\cot (\theta)$ equals $\square$





