Questions: Describe the sampling distribution of hatp based on large samples of size n-that is, given the mean, the standard deviation, and the (approximate) shape of the distribution of hatp when large samples of size n are (repeatedly) selected from the binomial distribution with a probability of success p. Let q=1-p.

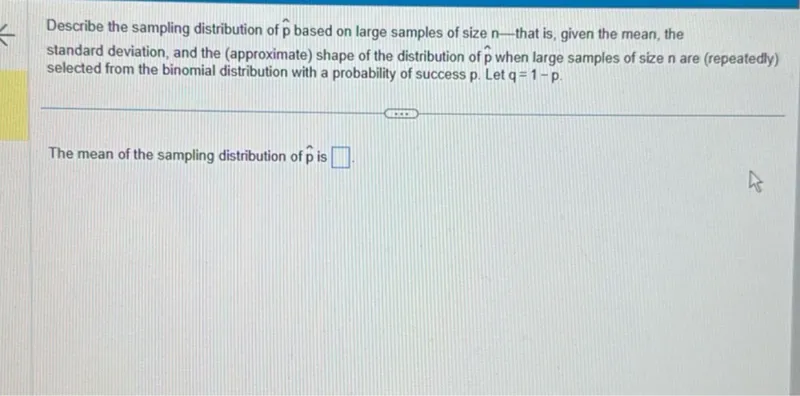
Describe the sampling distribution of hatp based on large samples of size n-that is, given the mean, the standard deviation, and the (approximate) shape of the distribution of hatp when large samples of size n are (repeatedly) selected from the binomial distribution with a probability of success p. Let q=1-p.

Transcript text: Describe the sampling distribution of $\hat{p}$ based on large samples of size $n$-that is, given the mean, the standard deviation, and the (approximate) shape of the distribution of $\hat{p}$ when large samples of size $n$ are (repeatedly) selected from the binomial distribution with a probability of success p . Let $\mathrm{q}=1-\mathrm{p}$.

Solution

Solution Steps

Step 1: Understanding the Sampling Distribution of $\hat{p}$

The sampling distribution of $\hat{p}$ refers to the distribution of the sample proportion $\hat{p}$ when large samples of size $n$ are repeatedly drawn from a binomial distribution with a probability of success $p$. Here, $\hat{p}$ is the proportion of successes in the sample.

Step 2: Calculating the Mean of the Sampling Distribution of $\hat{p}$

The mean of the sampling distribution of $\hat{p}$ is equal to the population proportion $p$. This is because, on average, the sample proportion $\hat{p}$ will be equal to the true population proportion $p$. Mathematically, this is expressed as: \[ \mu_{\hat{p}} = p \]

Step 3: Calculating the Standard Deviation of the Sampling Distribution of $\hat{p}$

The standard deviation of the sampling distribution of $\hat{p}$ is given by: \[ \sigma_{\hat{p}} = \sqrt{\frac{pq}{n}} \] where $q = 1 - p$. This formula accounts for the variability in the sample proportion due to the sample size $n$ and the population proportion $p$.

Step 4: Describing the Shape of the Sampling Distribution of $\hat{p}$

For large sample sizes $n$, the sampling distribution of $\hat{p}$ is approximately normal. This is due to the Central Limit Theorem, which states that the distribution of sample proportions will approach a normal distribution as $n$ increases, provided that $np$ and $nq$ are both greater than or equal to 5.