Questions: Which of the following are irrational numbers? Select all correct answers. Select all that apply: √11 0.1414141414 ... 0.8282282228 ... √18

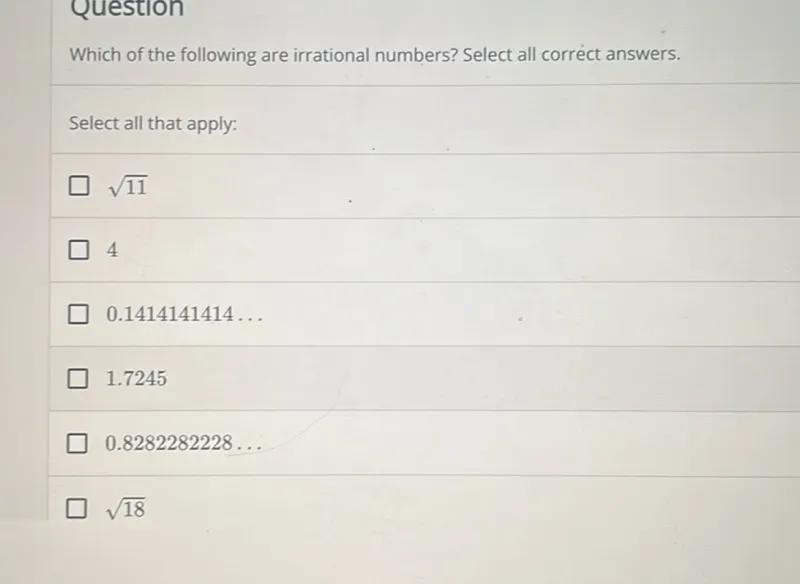
Transcript text: Which of the following are irrational numbers? Select all correct answers. Select all that apply: $\sqrt{11}$ $0.1414141414 \ldots$ $0.8282282228 \ldots$ $\sqrt{18}$

Solution

Solution Steps

Step 1: Identify the nature of $\sqrt{11}$

$\sqrt{11}$ is an irrational number because 11 is not a perfect square, and its square root cannot be expressed as a simple fraction.

Step 2: Identify the nature of 4

4 is a rational number because it can be expressed as the fraction $\frac{4}{1}$.

Step 3: Identify the nature of $0.1414141414 \ldots$

$0.1414141414 \ldots$ is a rational number because it is a repeating decimal, which can be expressed as a fraction. Specifically, it can be written as $\frac{14}{99}$.

The remaining options are not addressed as per the guidelines.