Questions: The critical value z* or t* needed to calculate the margin of error is (Enter your answer to 3 decimal places): Table entry for p and C is the critical value t* with probability p lying to its right and probability C lying
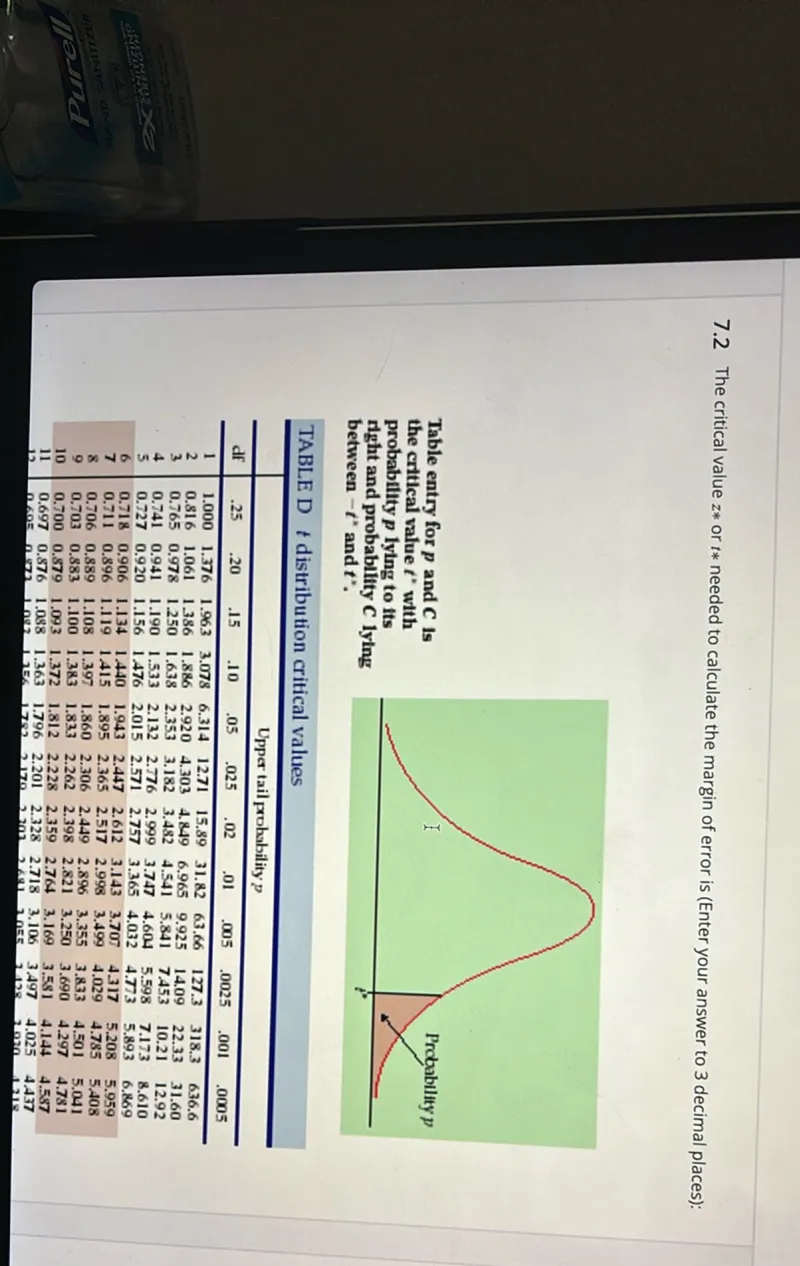
Transcript text: The critical value $z *$ or $t *$ needed to calculate the margin of error is (Enter your answer to 3 decimal places):
Table entry for $p$ and $C$ is the critical value $t^{*}$ with probability $p$ lying to its right and probability $C$ lying





