Questions: The area of a rectangle is 35 ft², and the length of the rectangle is 8 ft less than three times the width. Find the dimensions of the rectangle. Length : Width :

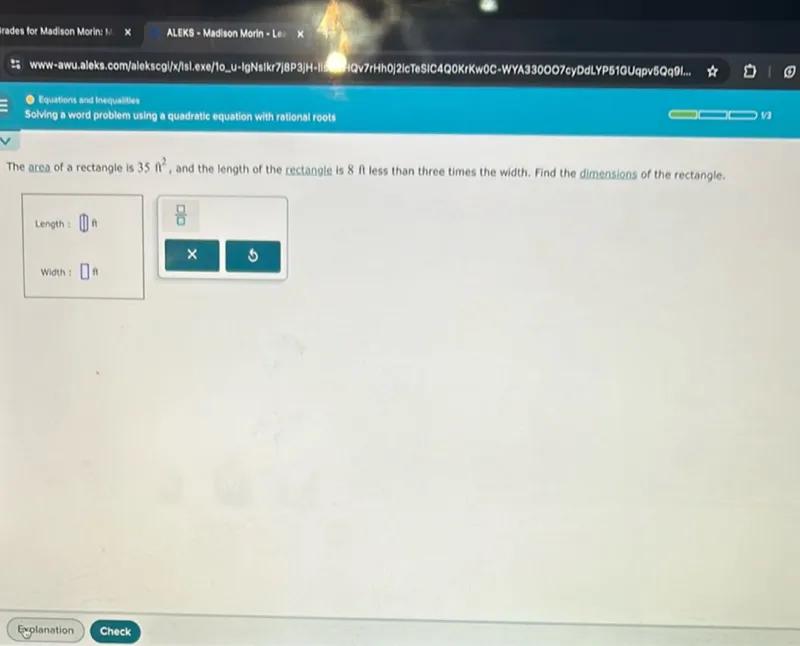
The area of a rectangle is 35 ft², and the length of the rectangle is 8 ft less than three times the width. Find the dimensions of the rectangle.

Length :

Width :

Transcript text: The area of a rectangle is $35 \mathrm{ft}^{2}$, and the length of the rectangle is 8 ft less than three times the width. Find the dimensions of the rectangle. Length : $\square$ Width : $\square$

Solution

Find the width of the rectangle.

Set up the equation for the area.

The area of the rectangle is given by $ w \times (3w - 8) = 35 $.

Solve the equation for $ w $.

The solutions to the equation are $ w = -\frac{7}{3} $ and $ w = 5 $. Since width must be positive, we take $ w = 5 $.

The width of the rectangle is \$\boxed{w = 5}\$.

Find the length of the rectangle.

Use the width to calculate the length.

The length is given by $ l = 3w - 8 $. Substituting $ w = 5 $, we find $ l = 3(5) - 8 = 15 - 8 = 7 $.

The length of the rectangle is \$\boxed{l = 7}\$.

The dimensions of the rectangle are: Width: \$\boxed{5}\$ ft Length: \$\boxed{7}\$ ft.

Was this solution helpful?

Unhelpful

Helpful

Questions asked by other users

< Previous Next >

Thank you for your feedback.

Copied!