Questions: Use the graph of f to state the value of each quantity, If it exists. If infinite, specify e a. lim x -> -4^- f(x) = □ b. lim x -> -2 f(x) = □ c. f(-2) = □ d. lim x -> 1^+ f(x) = □ e. lim x -> -1 f(x) = □ f. lim x -> 3^- f(x) = □ g. lim x -> 3^+ f(x) = □ h. f(3) = □ i. lim x -> -∞ f(x) = □ j. lim x -> ∞ f(x) = □

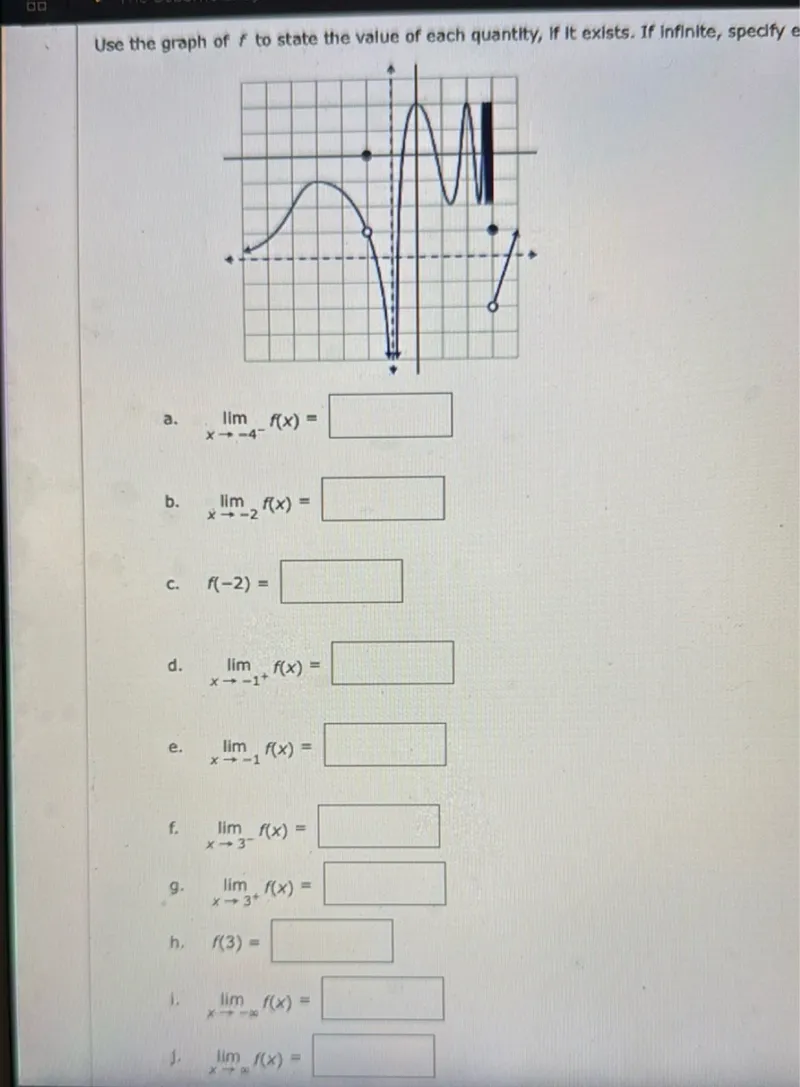
Use the graph of f to state the value of each quantity, If it exists. If infinite, specify e
a. lim x -> -4^- f(x) = □
b. lim x -> -2 f(x) = □
c. f(-2) = □
d. lim x -> 1^+ f(x) = □
e. lim x -> -1 f(x) = □
f. lim x -> 3^- f(x) = □
g. lim x -> 3^+ f(x) = □
h. f(3) = □
i. lim x -> -∞ f(x) = □
j. lim x -> ∞ f(x) = □

Transcript text: Use the graph of $f$ to state the value of each quantity, If it exists. If infinite, spedify e a. $\lim _{x \rightarrow-4^{-}} f(x)=$ $\square$ b. $\quad \lim _{x \rightarrow-2} f(x)=$ $\square$ c. $f(-2)=$ $\square$ d. $\lim _{x \rightarrow-1^{+}} f(x)=$ $\square$ e. $\lim _{x \rightarrow-1} f(x)=$ $\square$ f. $\lim _{x \rightarrow 3^{-}} f(x)=$ $\square$ g. $\lim _{x \rightarrow 3^{+}} f(x)=$ $\square$ h. $f(3)=$ $\square$ i. $\lim _{x \rightarrow-\infty} f(x)=$ $\square$ j. $\lim _{x \rightarrow \infty} f(x)=$ $\square$

Solution

Solution Steps

Step 1: Limit as x approaches -4 from the left

We observe the graph of $f(x)$ as $x$ approaches $-4$ from the left. The graph appears to approach the value $y=2$.

Step 2: Limit as x approaches -2

We observe the graph of $f(x)$ as $x$ approaches $-2$ from both the left and right. The graph approaches the value $y=1$.

Step 3: Value of f(-2)

We observe the graph of $f(x)$ at $x=-2$. There is an open circle at $(-2, 1)$ and a closed circle at $(-2, 2)$. The value of $f(-2)$ is given by the closed circle, so $f(-2) = 2$.