Questions: x f(x) 1 7 2 11 3 15 4 19 What is the function rule for the table above?

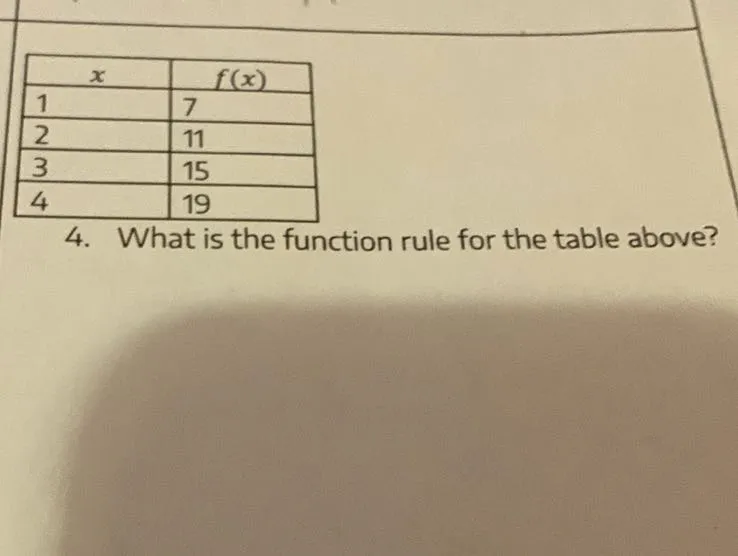
Transcript text: \begin{tabular}{|l|l|} \hline \multicolumn{1}{|c|}{$x$} & \multicolumn{1}{c|}{$f(x)$} \\ \hline 1 & 7 \\ \hline 2 & 11 \\ \hline 3 & 15 \\ \hline 4 & 19 \\ \hline \end{tabular} 4. What is the function rule for the table above?

Solution

Solution Steps

Step 1: Identify the pattern in the table

The table provides the following pairs of $ x $ and $ f(x) $:

$ x = 1 $, $ f(x) = 7 $
$ x = 2 $, $ f(x) = 11 $
$ x = 3 $, $ f(x) = 15 $
$ x = 4 $, $ f(x) = 19 $

Observe that as $ x $ increases by 1, $ f(x) $ increases by 4. This suggests a linear relationship.

Step 2: Determine the slope of the function

The slope $ m $ of a linear function $ f(x) = mx + b $ can be calculated using two points from the table. Using $ (1, 7) $ and $ (2, 11) $: \[ m = \frac{f(x_2) - f(x_1)}{x_2 - x_1} = \frac{11 - 7}{2 - 1} = \frac{4}{1} = 4 \]

Step 3: Find the y-intercept of the function

Using the slope $ m = 4 $ and one of the points, such as $ (1, 7) $, substitute into the equation $ f(x) = mx + b $: \[ 7 = 4(1) + b \] Solve for $ b $: \[ b = 7 - 4 = 3 \]

Step 4: Write the function rule

Substitute $ m = 4 $ and $ b = 3 $ into the linear equation: \[ f(x) = 4x + 3 \]