Questions: For the reaction NH4Cl(s) ⇌ NH3(g) + HCl(g) ΔH° = 176 kJ·mol⁻¹ and ΔG° = 91.2 kJ·mol⁻¹ at 298 K. What is the value of ΔG at 1000 K? (A) -109 kJ·mol⁻¹ (B) -64 kJ·mol⁻¹ (C) 64 kJ·mol⁻¹ (D) 109 kJ·mol⁻¹
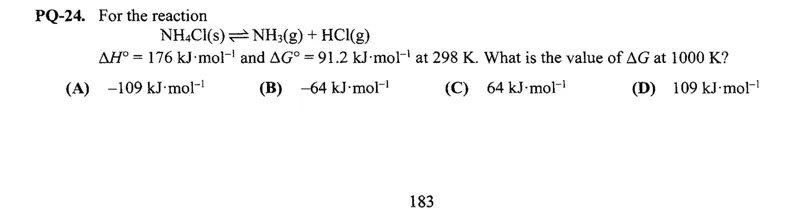
Transcript text: For the reaction
\[
\mathrm{NH}_{4} \mathrm{Cl}(\mathrm{~s}) \rightleftharpoons \mathrm{NH}_{3}(\mathrm{~g})+\mathrm{HCl}(\mathrm{~g})
\]
$\Delta H^{\circ}=176 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$ and $\Delta G^{\circ}=91.2 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$ at 298 K. What is the value of $\Delta G$ at 1000 K?
(A) $-109 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$
(B) $-64 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$
(C) $64 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$
(D) $109 \mathrm{~kJ} \cdot \mathrm{~mol}^{-1}$





