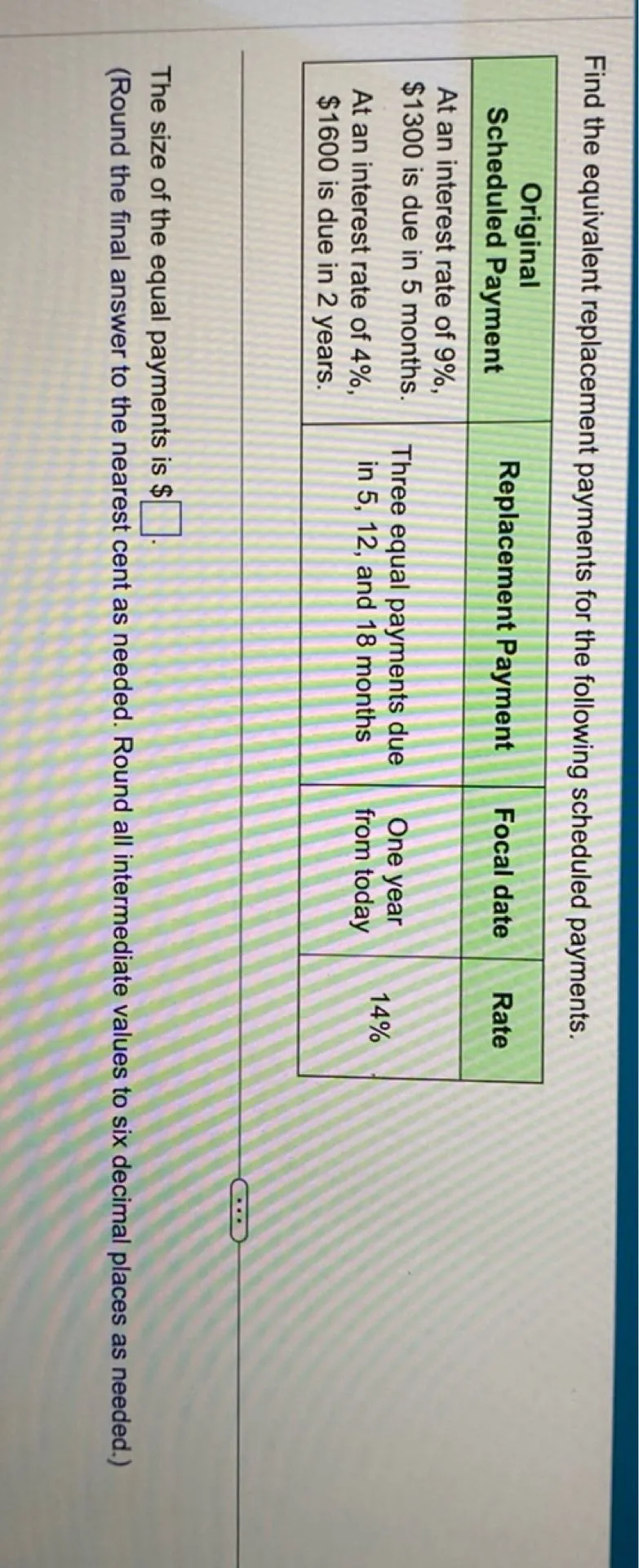
Find the size of the three equal replacement payments.
Calculate the future value of the \$1300 payment at the focal date.
The interest rate is 9% per year, so the monthly interest rate is \( \frac{0.09}{12} = 0.0075 \). The payment is due in 5 months, so it accumulates interest for \(12 - 5 = 7\) months. The future value of this payment at the focal date is calculated as:
\[1300(1 + 0.0075)^7\]
Calculate the future value of the \$1600 payment at the focal date.
The interest rate is 4% per year, so the monthly interest rate is \( \frac{0.04}{12} = 0.003333 \). The payment is due in 2 years (24 months), so we need to discount it back \(24 - 12 = 12\) months. The future value of this payment at the focal date is calculated as:
\[1600(1 + 0.003333)^{-12}\]
Calculate the future values of the three replacement payments at the focal date.
The interest rate is 14% per year, so the monthly interest rate is \( \frac{0.14}{12} = 0.011667 \).
- The first payment of \(X\) is due in 5 months, so it accumulates interest for \(12 - 5 = 7\) months. Its future value at the focal date is:
\[X(1 + 0.011667)^7\]
- The second payment of \(X\) is due in 12 months, so its future value at the focal date is:
\[X\]
- The third payment of \(X\) is due in 18 months, so we need to discount it back \(18 - 12 = 6\) months. Its future value at the focal date is:
\[X(1 + 0.011667)^{-6}\]
Set up the equation of value and solve for \(X\).
The equation of value is:
\[1300(1 + 0.0075)^7 + 1600(1 + 0.003333)^{-12} = X(1 + 0.011667)^7 + X + X(1 + 0.011667)^{-6}\]
Using Python or a calculator, solve for \(X\). The solution for \(X\) is approximately 963.485512. Rounding to the nearest cent, the size of the equal payments is:
\(\boxed{963.49}\)
The size of the equal payments is \(\boxed{963.49}\).
The size of the equal payments is \(\boxed{963.49}\).